TRİGONOMETRİ 3/6 SİN COS TEOREMİ VE ALAN FORMÜLÜ 11.SINIF 3/4 YouTube
Sinüs teoremi ve ispatı « Net Fikir
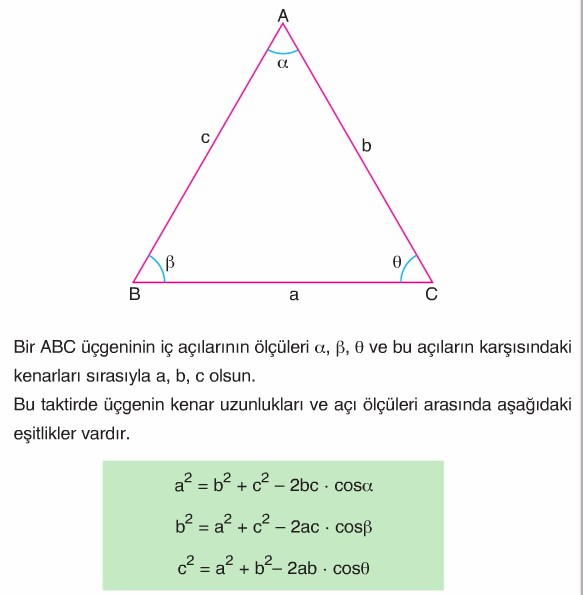
The sum and difference formulas allow expanding the sine, the cosine, and the tangent of a sum or a difference of two angles in terms of sines and cosines and tangents of the angles themselves.. This theorem can be proved by dividing the triangle into two right ones and using the Pythagorean theorem. The law of cosines can be used.

Sinüs teoremi nedir? Sin teoremi formülü ve açı hesaplama örnekleri ile konu anlatımı
The proof above requires that we draw two altitudes of the triangle. In the case of obtuse triangles, two of the altitudes are outside the triangle, so we need a slightly different proof. It uses one interior altitude as above, but also one exterior altitude. First the interior altitude. This is the same as the proof for acute triangles above.

TRİGONOMETRİ 3/6 SİN COS TEOREMİ VE ALAN FORMÜLÜ 11.SINIF 3/4 YouTube
From the definition of sine and cosine we determine the sides of the quadrilateral. The Law of Sines supplies the length of the remaining diagonal. The addition formula for sine is just a reformulation of Ptolemy's theorem. To prove the subtraction formula, let the side serve as a diameter. As a consequence, we obtain formulas for sine (in one.
Sinüs teoreminin ispatı özeldersci
The first four of these are known as the prosthaphaeresis formulas, or sometimes as Simpson's formulas. The sine and cosine angle addition identities can be compactly summarized by the matrix equation (7). Half-Angle Formulas, Harmonic Addition Theorem, Multiple-Angle Formulas, Prosthaphaeresis Formulas, Trigonometry Angles,.
11 Sınıf Matematik Trigonometri Konu Anlatımı Video
Law of Sines. Law of sines defines the ratio of sides of a triangle and their respective sine angles are equivalent to each other. The other names of the law of sines are sine law, sine rule and sine formula. The law of sine is used to find the unknown angle or the side of an oblique triangle. The oblique triangle is defined as any triangle.

Sinüs Teoremi Nedir? Sinüs Alan Formülü Nedir, Nasıl Hesaplanır? Son Dakika Eğitim Haberleri
Learn for free about math, art, computer programming, economics, physics, chemistry, biology, medicine, finance, history, and more. Khan Academy is a nonprofit with the mission of providing a free, world-class education for anyone, anywhere.

TRİGONOMETRİ TRİGONOMETRİK FONK. GRAFİKLERİ, COSSİN TEOREMİ YouTube
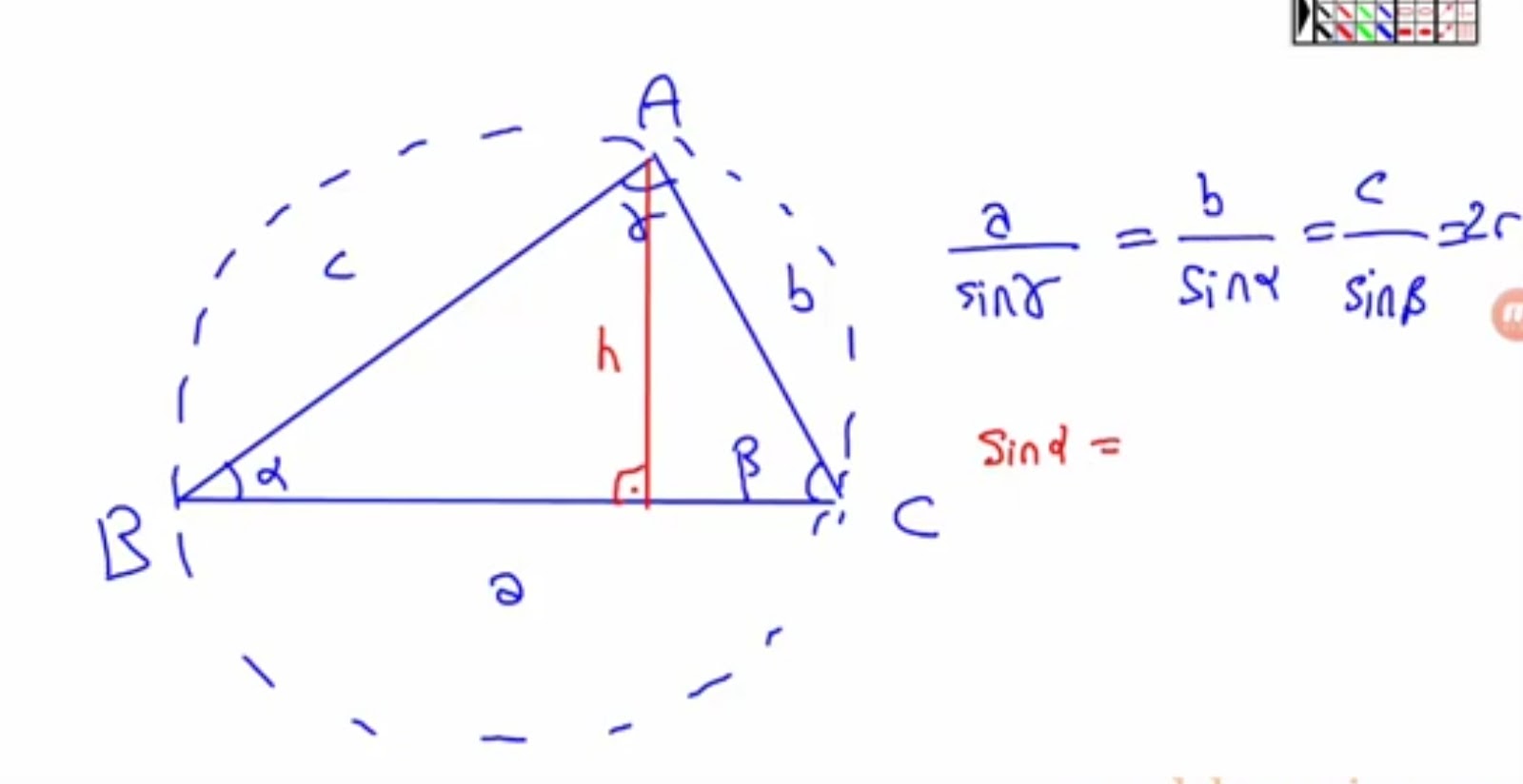
In trigonometry, the law of sines, sine law, sine formula, or sine rule is an equation relating the lengths of the sides of any triangle to the sines of its angles. According to the law, where a, b, and c are the lengths of the sides of a triangle, and α, β, and γ are the opposite angles (see figure 2), while R is the radius of the triangle.
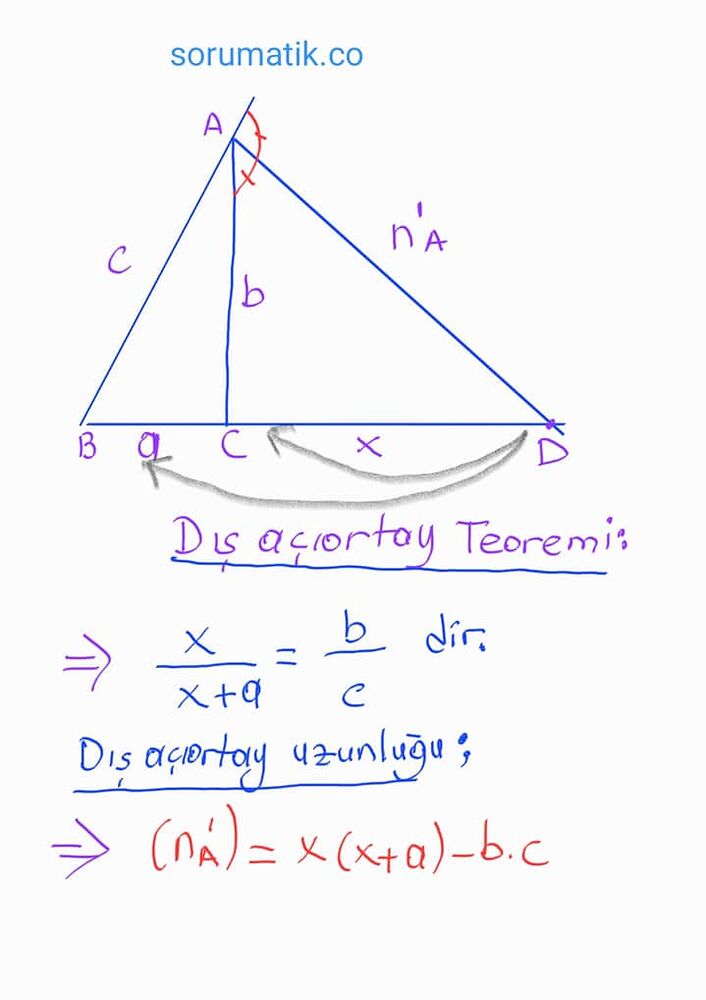
Dış açıortay Teoremi Formülü Nedir? Matematik Formülleri Sorumatik
The law of sines says that a / sin (30°) = b / sin (60°) = c / sin (90°). Now, you can express each of a,b,c with the help of any other of them. For instance, b and c expressed with the help of a read: c = 2 × a and b = √3 × a. Law of sines calculator finds the side lengths and angles of a triangle using the law of sines.
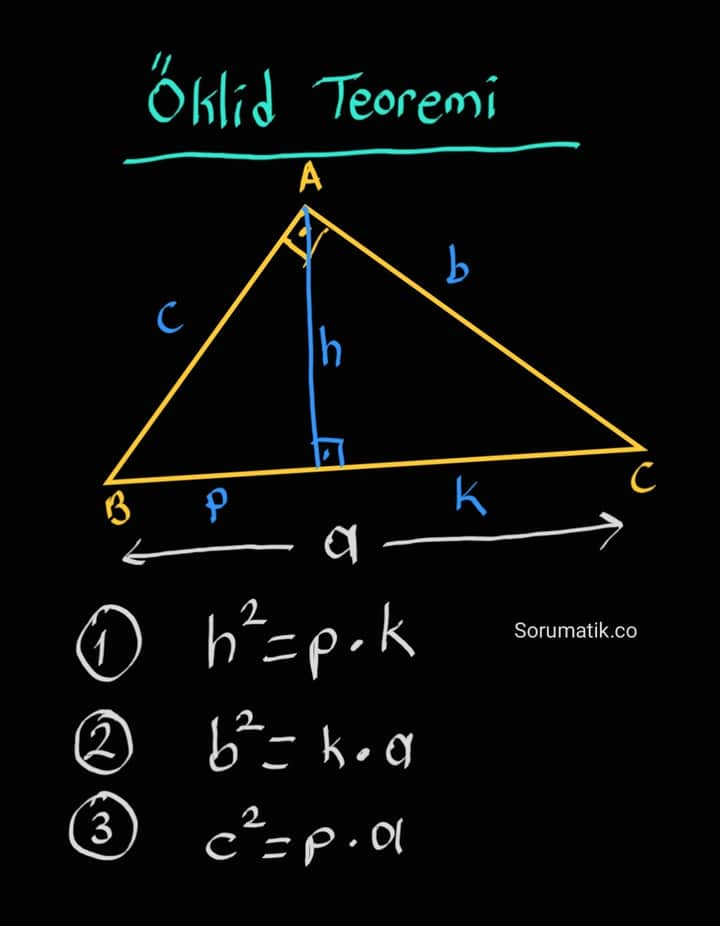
Öklid Teoremi Formülü nedir? (ispatı) Öklid bağıntısı kuralı YKS Geometri Sorumatik
Proofs and their relationships to the Pythagorean theorem Similar right triangles showing sine and cosine of angle θ Proof based on right-angle triangles. Any similar triangles have the property that if we select the same angle in all of them, the ratio of the two sides defining the angle is the same regardless of which similar triangle is selected, regardless of its actual size: the ratios.

TRİGONOMETRİ 3/6 PDF SİN COS TEOREMİ VE ALAN FORMÜLÜ 11.SINIF 3/4 YouTube
Using the Law of Sines, we get sin ( γ) 4 = sin (30 ∘) 2 so sin(γ) = 2sin(30 ∘) = 1. Now γ is an angle in a triangle which also contains α = 30 ∘. This means that γ must measure between 0 ∘ and 150 ∘ in order to fit inside the triangle with α. The only angle that satisfies this requirement and has sin(γ) = 1 is γ = 90 ∘.

Trigonometri Konu Anlatımı ve Örnek Soru Çözümü Kunduz
When those side-lengths are expressed in terms of the sin and cos values shown in the figure above, this yields the angle sum trigonometric identity for sine: sin(α + β) = sin α cos β + cos α sin β. Ptolemy's theorem is important in the history of trigonometric identities, as it is how results equivalent to the sum and difference formulas.
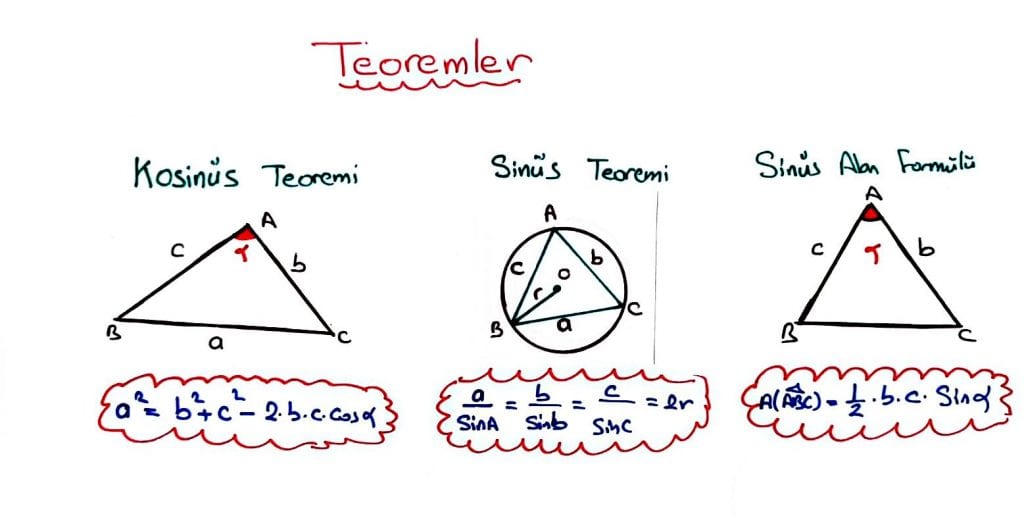
Trigonometri Alan Formuelue
The formula for the sine law is: a/sinA=b/sinB=c/sinC. a, b, c refer to the sides of any triangle, in no particular order. A, B, C are the angles opposite these sides respectively.
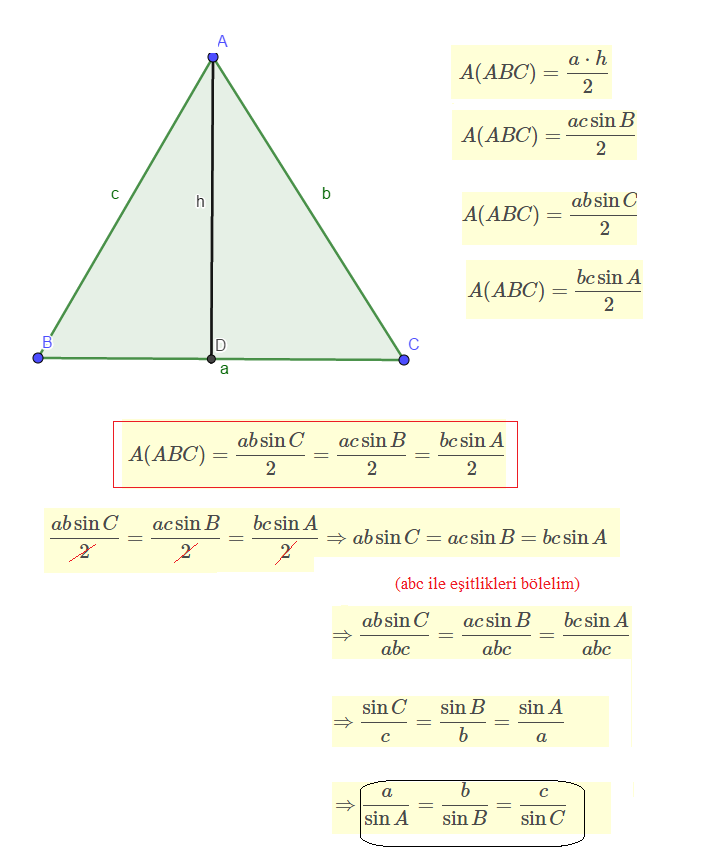
Sinüs teoremi ve ispatı « Net Fikir
The law of sines formula is used to relate the lengths of a triangle's sides to the sines of consecutive angles. It is the ratio of the length of the triangle's side to the sine of the angle formed by the other two remaining sides. Except for the SAS and SSS triangles, the law of sines formula is applied to any triangle.

trigonometri cossin teoremi 1 YouTube
sine, one of the six trigonometric functions, which, in a right triangle ABC, for an angle A, is sin A = length of side opposite angle A/ length of hypotenuse. (The other five trigonometric functions are cosine [cos], tangent [tan], secant [sec], cosecant [csc], and cotangent [cot].) From the definition of the cosine of angle A, cos A = length.

Trigonometri 13 (Sinüs Teoremi ve Sinüs Alan Formülü) YouTube
Sin teoremi formülü şu şekildedir: Bir üçgenin herhangi bir açısı θ'ye karşı, bu açının karşısındaki kenarın uzunluğunu a ifade edersek, sinθ = a/c şeklinde ifade edilir. Burada c, üçgenin hipotenüsünü veya en büyük kenarını temsil eder. Bu formül, trigonometri alanında sıklıkla kullanılan temel bir.
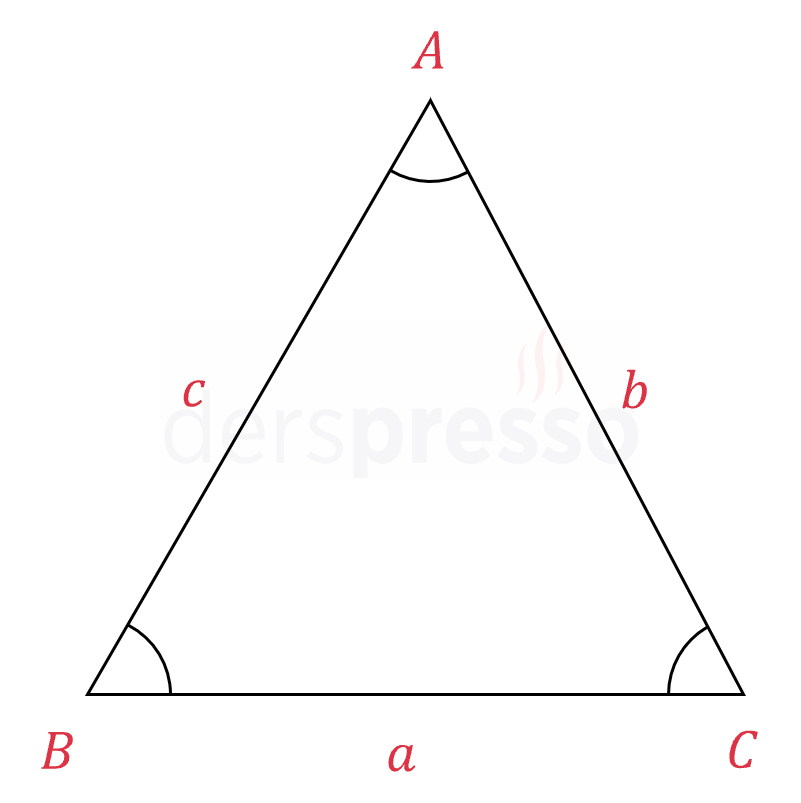
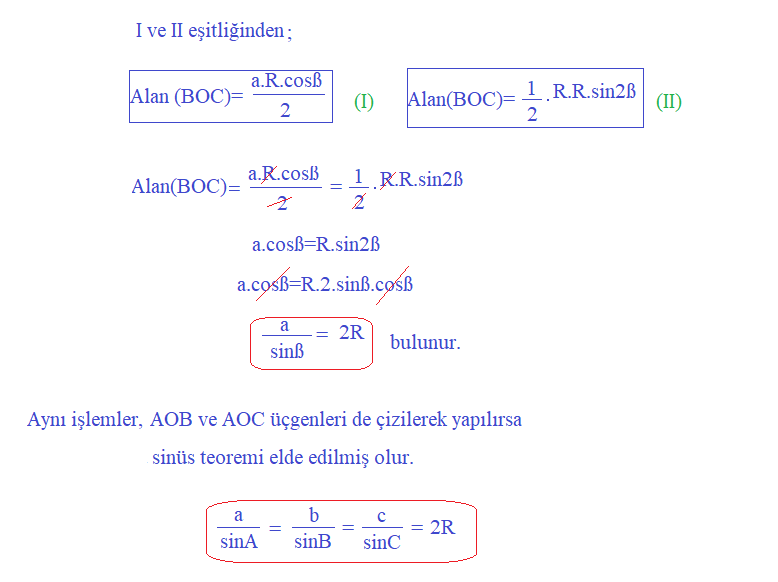
Kosinüs ve Sinüs Teoremleri
Multiple-Angle Formulas. For a positive integer, expressions of the form , , and can be expressed in terms of and only using the Euler formula and binomial theorem . where is the floor function . The function can also be expressed as a polynomial in (for odd) or times a polynomial in as. where is a Chebyshev polynomial of the first kind and is.